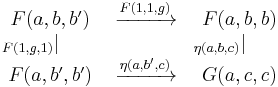Extranatural transformation
In mathematics, specifically in higher category theory, an extranatural transformation is a generalization of the notion of natural transformation.
Definition
Let  and
and  two functors of categories. A family
two functors of categories. A family 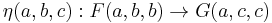 is said to be natural in a and extranatural in b and c if the following holds:
is said to be natural in a and extranatural in b and c if the following holds:
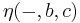 is a natural transformation (in the usual sense).
is a natural transformation (in the usual sense).- (extranaturality in b)
 ,
, 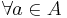 ,
, 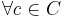 the following diagram commutes
the following diagram commutes
- (extranaturality in c)
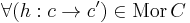 ,
, 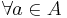 ,
, 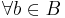 the following diagram commutes
the following diagram commutes